One thing I learned pretty early in life is that speakers are not meant to be wired together in a haphazard manner. In fact, whenever you plan to connect more than two speakers to a two-channel amplifier – or more than four speakers to a four-channel amp – there are a few things to consider, not the least of which is the amp’s ability to handle low-impedance loads. Ignoring the basics is like playing Russian roulette with your amplifier: If you’re lucky, it’ll drive the speakers without incident; if you’re not, the amp will fry.
The great thing about a multiple-speaker hookup is that once you master only two basic wiring procedures – “series” and “parallel” – the world is yours to conquer. When you know how many speakers you’re going to use and the impedance driving capability of your amplifier, you’ll be able to select a wiring scheme that will deliver the best sonic and electrical results. In some cases, it may not be one procedure or the other but a combination of the two that works best.
Speakers in Series
The essence of series wiring is really quite simple: When speakers are connected in this fashion, load impedance increases – the more speakers, the higher the impedance. The most common reason for wanting to raise impedance is to lower acoustical output, as in the case of rear-fill or center-channel speakers. Speaker output declines because the amplifier’s power output decreases as the load impedance increases. While you can connect any number of speakers in series, try to keep the total equivalent-load impedance for each channel below 16 ohms, since most amps are not designed to handle higher loads.
Figure 1A demonstrates how to wire a pair of speakers in series. The positive output terminal from one channel of the amplifier is wired to the positive terminal of Speaker A, and the negative terminal of Speaker A is connected to the positive terminal of Speaker B. Finally, a loop is created by wiring the negative terminal of Speaker B to the negative-output terminal of the same amplifier channel. The second channel is wired the same way.
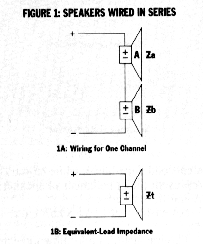
If you’re wiring more than two speakers in series, you simply continue alternating the negative and positive wires between speakers. To wire four speakers in series, for example, you connect the negative terminal of Speaker B to the positive terminal of Speaker C (instead of back to the amp); the negative terminal of that speaker is then wired to the positive terminal of Speaker D, and the loop is completed by connecting the negative terminal of Speaker D to the amp’s negative-output terminal.
To calculate the load impedance for the series-wired channel in Figure 1A, add up the impedances of each speaker in the chain. You can visualize the result as a single imaginary speaker (Figure 1B), whose impedance is represented by Zt. The math involves a simple equation in which Zt stands for the equivalent-load impedance and Za and Zb represent the impedances of Speakers A and B, respectively:
Equation 1: Speakers in Series
Zt = Za + Zb
Consider this real-world example of series wiring. Say you have a yearning for ultra-low bass – the kind that loosens the weather stripping around your windows – and you’re determined to install four 15-inch subwoofers in your car. The amplifier you’ve reserved for this task delivers 100 watts x 2 into 4 ohms and is capable of driving a minimum load impedance of 4 ohms; the subs are rated at 4 ohms apiece.
Assuming there’s enough room in your car for these monsters, the only viable option – given the above scenario – is to wire two subs in series to each amplifier channel. Doing so raises the net, or equivalent-load, impedance of each channel to 8 ohms – well within our standard 16-ohm ceiling. Mathematically, you substitute 4 ohms (the impedance rating of each sub) for Za and Zb in Equation 1 and work it through as follows:
Zt = Za + Zb
Zt = 4 + 4
Zt = 8 ohms
Parallel wiring, which we’ll discuss later, isn’t advisable here because the net impedance for each channel drops below the minimum-load rating of the amplifier.
Power Calculations
Whenever you connect more than one speaker to an amp channel, it’s important to gauge what effect the speakers will have on the amp and each driver in the chain. In other words, how much power will the amp deliver into each channel given the equivalent-load impedance you’ve created? And how much power will each speaker in the chain receive? Answering these questions will help you to avoid costly damage to your amp and speakers.
Referring back to the hypothetical subwoofer installation outlined above, we know that the amplifier in question is rated to deliver 100 watts x 2 into 4 ohms. To find out how much power each channel of this amplifier will deliver into the resulting 8-ohm load, we must solve Equation 2, in which Po is power output, Pr is the amp’s rated power, Zr is the impedance the amp’s output power is rated at, and Zt is the equivalent-load impedance for each channel:
Equation 2: Calculating Output Power
Po = Pr x (Zr / Zt)
Plugging in the appropriate numbers, the calculation is worked through as follows:
Po = 100 x (4 / 8)
Po = 100 x 0.5
Po = 50 watts
Now that we know each amplifier channel will deliver 50 watts into an 8-ohm load, we can figure out how much power will be applied to one of the subwoofers – Pa – by solving Equation 3, in which Zn stands for the rated impedance of the speaker:
Equation 3: Power Applied to Each Driver
Pa = Po x (Zn / Zt)
Substituting 50 for Po, 4 for Zn, and 8 for Zt, the equation works through as follows:
Pa = 50 x (4 / 8)
Pa = 50 x 0.5
Pa = 25 watts
Since both subwoofers are rated at 4 ohms, we know that the second subwoofer (Pb) would also receive 25 watts.
Speakers in Parallel
Parallel wiring has the opposite effect of series wiring – load impedance drops when speakers are wired in this fashion. And the more speakers you wire in, the lower the impedance. The most common reason for wanting to lower impedance is to raise acoustical output. Speaker output increases because the amplifier’s power output rises as the load impedance decreases.
The number of speakers that can be connected in parallel is limited by the minimum load impedance that the amplifier is capable of driving and the power-handling capacity of the speakers. In most cases, load impedance should be held to a minimum of 2 ohms – provided the amplifier can handle impedances that low.
Figure 2A shows how to wire a pair of speakers in parallel. A wire from the positive terminal of one channel of the amp is wired to the positive terminals on speakers A and B. (The simplest way to do this is to run a wire from the amp terminal to Speaker A and then run a second wire from that terminal to Speaker B.) Then the negative terminal of the same amp channel is wired in like fashion to the negative terminals on both speakers. The second channel is wired the same way.
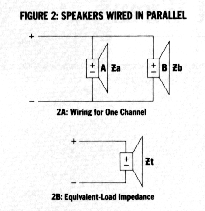
Calculating the load impedance for the parallel-wired channel in Figure 2A is a bit more complicated than doing so for speakers wired in series. Using Equation 4, multiply the impedances of each speaker and then divide the result by the sum of the speakers’ impedances. You can visualize the result as a single imaginary speaker (Figure 2B), whose impedance is represented by Zt. Zt stands for the equivalent-load impedance, while Za and Zb represent the impedances of speakers A and B, respectively.
Equation 4: Speakers in Parallel
Zt = (Za x Zb) / (Za + Zb)
Turning again to our subwoofer install, say you want even more oomph from your system. So you trade in the original amp for one that has the same 4-ohm power rating (100 watts x 2) but is also 2-ohm stable. Since the power output of most amps increases as impedance decreases, you could boost the amp’s power output and the system’s bass response simply by switching to a parallel wiring scheme. Doing so would drop the net, or equivalent-load, impedance for each channel to 2 ohms. Mathematically, you substitute 4 for Za and Zb in Equation 4 and work it through:
Zt = (Za x Zb) / (Za + Zb)
Zt = (4 x 4) / (4 + 4)
Zt = 16 / 8
Zt = 2 ohms
To calculate the new amplifier’s power output into 2 ohms, refer to Equation 2. Plugging in the appropriate numbers, the calculation goes as follows:
Po = 100 x (4 / 2)
Po = 100 x 2
Po = 200 watts
As you can see, by upgrading to a 2-ohm-stable amplifier and wiring the same four 15-inch woofers in parallel – two per channel – power output jumps fourfold – from 50 watts x 2 to 200 watts x 2.
Now, to find out how much power each subwoofer will receive when wired in parallel, we must use Equation 5, which is actually a scrambled version of Equation 3 (remember, we’ll be working the equation for just one speaker (Pa)):
Equation 5: Power Applied to Each Speaker
Pa = Po x (Zt / Zn)
Substituting 200 for Po, 2 for Zt, and 4 for Zn, the equation works through as follows:
Pa = 200 x (2 / 4)
Pa = 200 x 0.5
Pa = 100 watts
Since both subwoofers are rated at 4 ohms, the second one (Pb) would also receive 100 watts.
Series/Parallel Wiring
Now it’s time to combine the two wiring methods. The most common reason for wanting to do this is to increase the number of speakers you can use in your system – perhaps to achieve greater volume and/or visual effect – and still maintain an impedance load that’s compatible with the system’s amplifier. Any number of speakers can be linked using a series/ parallel wiring scheme, as long as you keep the total equivalent-load impedance between 2 and 16 ohms.
Figure 3A shows how to wire four speakers to a single channel using a typical series/parallel combination. A single wire running from the amp’s positive terminal runs to the positive terminals of speakers A and C. Next, the negative terminals of Speakers A and C are wired to the positive terminals of Speakers B and D, respectively. Finally, a loop is created by running a single wire from the negative terminal of the amp channel and splitting it between the negative terminals of Speakers B and D.
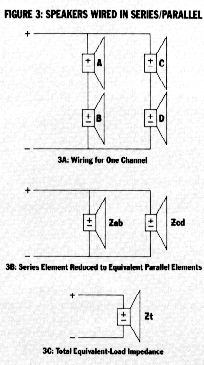
The best way to understand the electrical implications of this wiring scheme is to conceptualize it in three stages, as represented by Figures 3A, 3B, and 3C. First, draw the entire wiring scheme for one channel on paper, following Figure 3A. Next, simplify the diagram by replacing each pair of series-wired speakers – A/B and C/D – with an imaginary equivalent speaker, as shown in Figure 3B. We’ll call these “combined” drivers Zab and Zcd. Now, reduce these speakers to a single, equivalent driver and call it Zt (Figure 3C). In a nutshell, we have reduced a relatively complex four-speaker system down to one imaginary driver, which represents the total load impedance created by wiring four speakers in a series/parallel combination.
Calculating the load impedance of the series/parallel-wired channel in Figure 3A is a three-step process. First use Equation 1 (Zt = Za + Zb) to find the equivalent-load impedance of Speakers A and B, which are wired in series. Then repeat the process for speakers C and D, changing the variables in Equation 1 (Zt = Zc + Zd). Finally, to find a single, total equivalent-load impedance for the “combined” speakers Zab and Zcd, substitute new variables into Equation 4 [Zt = (Za x Zb) / (Za + Zb) becomes Zt = (Zab x Zcd) / (Zab + Zcd)].
To work through this series of equations, we’ll take our hypothetical subwoofer installation yet another step further. Say the four subwoofers wired in parallel to your new 2-ohm amplifier are no longer good enough. So you buy four more subs – that’s a total of eight. Just to show that it can be done, you decide to stick with the same 2-ohm-stable amplifier, which is rated at 100 watts x 2 into 4 ohms; the new subs are also rated at 4 ohms apiece.
Now what? You could wire four speakers in series to each channel, but this would yield a 16-ohm load. Another option is to wire four speakers in parallel to each channel, but this would yield a dangerously low 1-ohm load. The only practical option, therefore, is to combine the two wiring methods in accordance with Figure 3A. First, you connect two subwoofers in series and then wire that pair in parallel to a second pair, which is also connected in series. Follow the same procedure for the other channel.
The first step in determining the total equivalent-load impedance for each channel is to plug the appropriate impedance values into Equation 1 and work it through for each series-wired speaker pair, A/B and C/D. Plug in the values for speakers A and B and solve Equation 1 as follows:
Zt = Za + Zb
Zt = 4 + 4
Zt = 8ohms
Then repeat the calculation using speakers C and D. Since each of the speakers is rated at 4 ohms, the equivalent-load impedance for each series-wired speaker pair is 8 ohms.
Redraw the circuit diagram and replace speakers A and B with Zab to represent the new equivalent-load impedance. Do the same for speakers C and D to create Zcd. The result should be a one-channel diagram that resembles Figure 3B, with the label “8 ohms” in place of Zab and Zcd.
The next step is to find the total equivalent-load impedance of the channel by plugging the new 8-ohm values for Zab and Zcd into Equation 4, as follows:
Zt = (Zab x Zcd) / (Zab + Zcd)
Zt = (8 x 8) / (8 + 8)
Zt = 64 / 16
Zt = 4 ohms
Sketch a new one-channel diagram showing the total equivalent-load impedance. The drawing should look like Figure 3C, with the label “4 ohms” in place of Zt.
Now we know that four speakers connected to one amplifier channel with series/parallel wiring creates a 4-ohm load. Since the amplifier is rated to deliver 100 watts x 2 into a 4-ohm load, we know that each channel will receive 100 watts. To verify this, we can plug the appropriate numbers into Equation 2 and work it through as follows:
Po = Pr x (Zr / Zt)
Po = 100 x (4 / 4)
Po = 100 x 1
Po = 100 watts
As anticipated, each amplifier channel will pump out 100 watts.
To find out how much power each series-wired speaker pair (equivalent-load speakers Zab and Zcd) will receive, plug in the appropriate numbers and solve Equation 5. We use this equation because Zab and Zcd are parallel-wired to one another. Working with Zab, substitute 100 for Po, 4 for Zt, and 8 for Zab. The calculation goes as foliows:
Pab = Po x (Zt / Zab)
Pab = 100 x (4 / 8)
Pab = 100 x 0.5
Pab = 50 watts
The math for Zcd is identical, since both speakers are rated at 4 ohms, so Zab and Zcd each receive 50 watts of power.
But Zab and Zcd are imaginary drivers, each of which represents a series-wired speaker pair. To figure out how much power each real speaker will receive, work through Equation 3, substituting 50 for Po (the amplifier’s output power into Zab and Zcd), 4 for Zn (the speaker’s rated impedance), and 8 for Zt (the equivalent impedance of Zab and Zcd). The calculation goes as follows:
Pn = Po x (Zn / Zt)
Pn = 50 x (4 / 8)
Pa = 50 x 0.5
Pa = 25 watts
Technically, you need to repeat this process for each driver – B, C, and D – but since each driver in our example is rated at 4 ohms, you’ll get the same results.
Crossovers
Virtually all multi-speaker installations use at least one passive crossover. We’ve ignored crossovers up to this point because they have a nasty habit of confounding things. There are two key points to remember when passive crossovers are introduced into the picture: First, the crossover must be matched – impedance-wise and, when a custom network is involved, in the values of its capacitors and inductors – to the equivalent-load impedance of the drivers. Secondly, the impedance of an amplifier channel that employs passive crossovers can be calculated only for a specific frequency that falls within the crossover’s passband.
Passive crossovers affect the load impedance “seen” by the amplifier, but the effect varies from frequency to frequency. For frequencies that fall within the crossover’s passband, the crossover’s impedance is very low – for practical purposes, zero. This means that you can forget about the crossover when dealing with frequencies within its passband. For all other frequencies, the crossover’s impedance rises, and the farther the frequency falls outside of the passband, the higher the crossover’s impedance.
Returning once again to our subwoofer saga, say you’ve grown tired of your eight subs and now want to design a system that focuses on sound quality – not quantity. So you design a speaker system modeled on the one laid out in Figure 4 (one channel shown). The system comprises two 10-inch woofers, pairs of front and rear 5-inch midranges, and pairs of front and rear soft-dome tweeters. All of the speakers are rated at 8 ohms and are wired in parallel – except the subs, which are wired in parallel but have a 4-ohm rating. A low-pass crossover sends signals below 100 Hz to the woofers, a band-pass crossover allows the midranges to play between 100 and 6,000 Hz, and the high-pass crossover sends signals above 6,000 Hz to the tweeters.
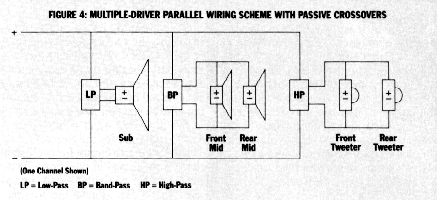
Since it’s impossible to come up with a single load-impedance (Zt) figure for the above channel configuration (since impedance varies with frequency), we’ll examine what happens to the load at three different frequencies – 50, 500, and 10,000 Hz. The first step in answering this question is to simplify the circuit layout by replacing the two parallel-wired midranges in each channel with a single imaginary speaker that has an equivalent impedance of 4 ohms. (4 ohms is established by plugging the 8-ohm rat- ing of each speaker into Equation 4)
Zt = (8 x 8) / (4 + 4)
Zt = 64 / 8
Zt = 4
Do the same for the tweeters.
Next, sketch a circuit for each of the three frequencies in question. To determine the equivalent-load impedance at 50 Hz, delete the low-pass crossover from the original drawing, since 50 Hz is within the passband of that device. 50 Hz falls outside of the passband for the band-pass and high-pass crossovers, however, so eliminate the midranges and tweeters from the diagram. What you wind up with is a single 4-ohm woofer with a positive and negative lead running to it. It follows, then, that the amplifier will see an equivalent-load impedance (Zt) of 4 ohms at 50 Hz.
Since 500 Hz is within the passband of the midrange crossover, begin the 500-Hz sketch by excluding the crossover. This isn’t the case for the low- and high-pass crossovers, however, so eliminate the woofer and tweeters from the original circuit diagram and redraw the circuit as a single imaginary midrange speaker with an equivalent-load impedance of 4 ohms. All of this boils down to the fact that the power amplifier will see a total equivalent-load impedance (Zt) of 4 ohms at 500 Hz.
Begin the 10,000-Hz drawing by eliminating the woofer and midranges, since this frequency falls outside of the passband for the low- and band-pass crossovers. Next, redraw the circuit as a single imaginary tweeter with an equivalent-load impedance of 4 ohms. The bottom line: The amplifier will see a total equivalent-load impedance (Zt) of 4 ohms at 10,000 Hz.
The consistent 4-ohm findings in these exercises indicate that impedance will remain fairly constant – at about 4 ohms – throughout the musical spectrum.
Pulling It All Together
By now, you should have a pretty good feel for the fundamentals of multi-speaker wiring (or a bad head- ache). When designing a system on your own, don’t forget the minimum-load impedance rating of the amp you plan to use. If the manufacturer rates it at 2 ohms, leave it at that – don’t bother creating a 1-ohm load unless you’re fascinated by pyrotechnics. And note that the amp in systems incorporating low-impedance loads tends to lose its ability to control or “dampen” unwanted speaker-cone movement. The result is bass that’s “muddy” or distorted.
Reliability also is a concern. Car amplifiers tend to be only about 50-percent efficient, which means that half of all the power they use is converted into heat. In other words, as power output increases, so does the amplifier’s operating temperature; if the amp gets too hot, it may shut down. And when it comes to claims of low-impedance stability, keep in mind that how long the amplifier can sustain output into a low-impedance load is very important. If an amp is rated to deliver 150 watts x 2 into 2 ohms but does so for only 5 minutes before its thermal-protection circuit kicks in, it won’t be of much use.
A concern regarding speaker impedance involves mixing speakers with different impedance ratings. Avoid doing this, because drivers with different impedances will “see” different amounts of power.
And last but not least, pay attention to polarity while you’re wiring up your masterpiece. Make sure each driver is correctly wired – positive amp terminal to positive speaker terminal, and so on – or cancellation problems will conspire to drive you nuts.
All of this may sound like an awful lot of trouble to go through just to wire a few speakers to your amplifier. And having just digested such a large chunk of information, it’s natural that you would feel that way. But once you hit that garage and begin tinkering into the late-night hours, you’ll find solace in knowing that even the most complex wiring schemes can be reduced to a simple sketch. Who knows, if you work extra hard at it you may just break my record for connecting the most drivers – thirty-two – to a single amplifier.
